By Lauriston de Sousa Trindade, Alfredo Dal’Ava Jr, Cristovão Jacques Faria, Marcelo Zurita, Gabriel Gonçalves Silva
Abstract: The Brazilian Meteor Observation Network, BRAMON, reports the discovery of two meteor showers, observed after a search in its own database. For the meteor shower #1042 SCP, one meteor was found in 2015, six meteors in 2016, four meteors in 2017, one meteor in 2018, one meteor in 2019 and three meteors in 2020, occurring between solar longitudes of 103º and 138º. The mean radiant position is at right ascension of 312.2º and declination of –20.8º. For the meteor shower #1043 OGS, the evidence is based on one meteor in 2014, one meteor in 2015, three meteors in 2016, eight meteors in 2017, two meteors in 2018 and five meteors in 2020, occurring between solar longitudes of 100º and 126º. The mean radiant position is at right ascension of 297.3º and declination of –26.7°. The asteroids 2019 OK and 2017 NT5 were identified as the most probable parent bodies of the particles responsible for the meteor showers.
1 Introduction
BRAMON is a meteor-monitoring network that was created in 2014 to record and study meteors over Brazil. The main goals of the network are the search for new meteor showers, as well as the work to provide more detailed information about very bright bolides and meteorite-dropping meteors (Zurita et al., 2019). Taking advantage of its large latitude coverage, extending from 2ºS to 30ºS, and privileged position in the southern hemisphere (Amaral et al., 2018), BRAMON accumulated thousands of meteor orbits over 7 years of activity. The collected data has allowed the search for meteor radiants and to update the already cataloged meteor showers with new orbits. As a result of this effort, it was possible to create BRAMON’s own meteor orbit database, made available along with the EDMOND’s database (Amaral et al., 2018).
The production of a large meteor database and the study of the meteor orbit patterns are the basis of the meteor shower studies.
For a long time, the meteor phenomenon was associated with atmospheric effects of terrestrial origin. This Aristotelian model remained in force until the beginning of the 19th century. In 1861 Schiaparelli discovered the asteroid Hesperia. Five years later he demonstrated that some meteors had orbits similar to some comets and concluded that the meteor showers are the remnants of comets. In particular, he calculated the comet 1862 III as parent body of the Perseids meteors and comet 1866 I as the source for the Leonids (Schiaparelli, 1867a; 1867b).
The relationship between comets and meteor showers was only widely accepted after the confirmation, in 1872, of the association of the intense meteor shower of the Andromedids with comet 3D/Biela, correctly predicted years before (Weiss, 1868).
Not only comets can be listed as parent bodies of a meteor shower. Many asteroids have been identified as having a similar orbit as the Taurids meteor shower (Clube and Napler, 1984). Since then, many other associations with meteor showers have emerged (Porubčan et al., 2004).
There are several examples of association between asteroids and meteor showers (Jopek and Williams, 2013): the Quadrantids (QUA) meteor shower is associated with the comet C/1490Y1 and the asteroid 2003 EH1 (Jenniskens, 2004; Williams et al., 2004; Micheli et al., 2008); the Geminids (GEM) meteor shower is associated with asteroid Phaethon (Whipple, 1983); and the Taurid complex is related with the comet P/Encke (Wipple and El-Din Hamid, 1952).
The origin of the meteor showers had been solved. But the perception was that meteoroid streams were not homogeneous in space. With systematic observations over many years, the fluctuations in the rates of the occurrences of meteors could be monitored. An example of this occurred with the Leonids. In 1833 there was a real meteor storm, but in the following years the ZHR (zenithal hourly rate) remained much lower, with cyclical fluctuations increasing the rates every 33 years.
Thus, the activity of meteor showers can be classified into two groups: annual showers, which are visible every single year within a defined date range and predictable activity rate, and the outbursts, in which the occurrence of a high rate of meteors in a given year does not necessarily happen again in the following years. Eventually an established annual shower may present an outburst, characterized by a noticeable increase in its meteor rates.
The outbursts can be seen as young trails, such as the ones often observed for the Leonid radiant, associated with comet 55P/Temple-Tuttle (Yeomans and Yau, 1996). More stable dust trails may be a sign of a well-established trail, making it possible to estimate the overall age of the meteoroid stream. This is the case for the Perseids and their parent comet 109P/Swift-Tuttle (Brown and Jones, 1998).
The current meteor science admits that meteoroid streams are formed by particles detached from comets and some asteroids, many of them being dormant comets. There are several mechanisms recognized to release the dust that forms the meteoroid streams: ejection, disintegration by impact, rotational instability, electrostatic repulsion, radiation pressure, dehydration stress, thermal fracture and sublimation of ice (Jewitt et al., 2015). Thus, the fragments disperse in their own orbits, but which can maintain similarity for many years or even centuries.
Considering that asteroids and dormant comets have a low ejection of particle matter and considering that the BRAMON database still has a few thousand meteor orbits, the best way to search for new meteor showers associated with asteroids would be to use the NEA asteroid list as an initial reference.
In this study, we describe the discovery of two new meteor showers from the southern hemisphere. During this work, different dissimilarity criteria were applied combined on the orbits to assure the validity of the new showers. The identification of the most probable parent body of each meteor shower was also possible using the same comparison methodology. The shower discovery was only possible thanks to the cooperation between BRAMON and SONEAR, a southern hemisphere observatory dedicated to near-Earth asteroids research.
2 Methodology
2.1 Dissimilarity Criteria
All objects in the Solar System can be characterized by their Keplerian orbital elements: a (semi-major axis), q (perihelion distance), e (eccentricity), i (inclination), ω (argument of perihelion) and Ω (longitude of the ascending node). For meteoroids that are part of the meteor showers, in addition to the Keplerian orbital elements, it is necessary to compare all these orbits using a similarity criterion. The most common way to measure similarity between orbits is by the concept of the so-called discriminant criterion or D-criterion. D-criterion methods seek to measure the distance between two N-dimensional points in space, using the orbital elements of orbits that are evaluated.
During the development of D-criteria, different authors implemented distinct mathematical expressions, using different numbers of orbital parameters. In this paper the authors used 5 orbital elements for the D-criteria as proposed by Southworth and Hawkins (1963), Drummond (1981) and Jopek (1993).
The Southworth and Hawkins D-criterion (DSH) has the following mathematic expression in Equation 1:
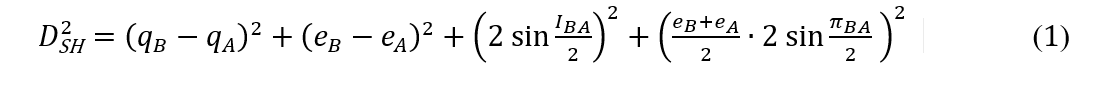
where eA and eB is the eccentricity, and qA and qB is the perihelion distance of two orbits, IBA is the angle between two orbital planes, and πBA is the distance of the longitudes of perihelia measured from the intersection of the orbits.
Drummond (1981), proposed some modifications in the dissimilarity criterion of Southworth & Hawkins (1963), resulting in Drummond D-Criterion (DD), for which the definition is shown in Equation 2:
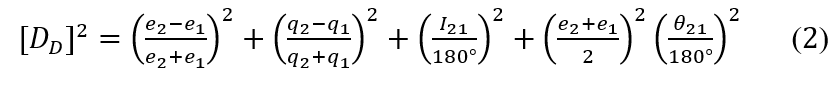
where, I21 is the angle between the two orbital planes and θ21 is the angle between the perihelion points of each orbit, defined in Equation 3:
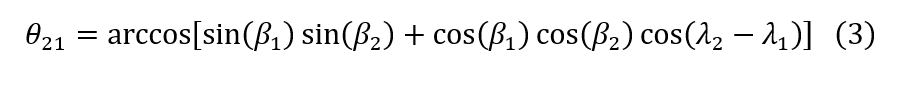
where, λ and β are respectively the longitude and ecliptic latitude of the perihelion defined in Equations 4 and 5:

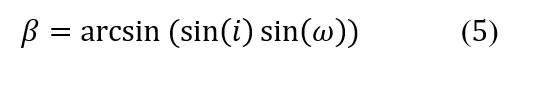
Jopek (1993) concluded that DSH depends a lot on the perihelion distance q and that DD depends a lot on the eccentricity e. Thus, he proposed a new method (DH), which has the following mathematical expression in Equation 6:
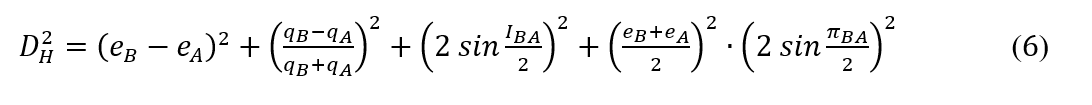
2.2 Meteor Shower Search
The search began with the selection of 32 asteroids discovered by SONEAR (Southern Observatory for Near Earth Asteroid Research) that had been classified as NEAs. We employed the orbital elements by the IAU Minor Planet Data Center, available at Epoch 2459200.5 (2020-Dec-17.0).
The orbit of each asteroid was used as input in a C++/Python calculator that tested each orbit of BRAMON’s database and returned the results within the thresholds established for DD, DSH and DH. The threshold was established as Dc (Maximum tolerated discrimination value) so that, if D(X, Y) < Dc, the orbits are sufficiently similar and the asteroid is a candidate to be associated with the tested meteor.
There are different methods to determine the threshold: Porubčan et al. (2006) opted for a DSH of 0.3 in the case of association of NEOs with the Taurid Complex; Rudawska et al. (2012a) established a DSH threshold of 0.084 and DH of 0.077 for the association of asteroids and meteor showers. Šegon et al. (2014) used a 0.15 threshold for both DSH and DH for the association between meteor showers and asteroids. In the search for meteors associated with the asteroids discovered by SONEAR we use the following thresholds: DD < 0.09, DSH < 0.15 and DH < 0.15.
2.3 Backward Integration
It is not possible to find a solution to the N-body problem, in an analytical way, if there are more than three gravitational interrelated massive bodies. If there are three bodies for the calculations, it is only possible to proceed analytically if one of these bodies can have the mass despised (Bhatnagar K.B. and Saha L.M., 1993).
Currently, there are several orbital integrators that solve the N-body problem. One of the classic integrators is the MERCURY hybrid symplectic integrator (Chambers, 1999). Non-symplectic integrators can preserve the amount of area in the solution of the N-body problem (Kinoshita et al., 1991). The choice of the integrator is particularly important because, during the integration, the sizes of timestep-variations can accumulate errors if there are too many close encounters in the time range chosen in the study.
The backward integration of the orbits of the asteroids and the mean orbit of the clusters of meteors found were proceeded using the Rebound package as integrator (Rein & Liu, 2012). Unlike MERCURY, Rebound has a more agile and user-friendly interface, using Python/C++ language and implements several integration algorithms. Another advantage of Rebound is that it has a module that can access the NASA JPL Horizons database directly through Internet. This gives the possibility to start backward simulations with the most accurate ephemeris data of massive bodies (barycenter) used in the study (Sun, Mercury, Earth, Moon, Mars, Ceres, Vesta, Jupiter, Saturn, Uranus and Neptune).
The initial studies were done using the integrator algorithm WHFAST (Rein and Tamayo, 2015) because it implements fast and accurate responses of a Wisdom-Holman integrator, allowing to run planetary system integrations of longer time ranges more quickly. Thus, the integration was done for 5000 years, with an initial timestep of 0.0007 days while capturing 500000 intermediate results for analysis. The objective of this verification was to compare the fluctuation of the orbital elements of multiple objects over time.
It is important to note that it is not possible to specify the ejection dates of meteoroids that have collided with the Earth’s atmosphere over the past few years simply using backwards integrations. For this, it would be necessary to associate models of non-gravitational disturbances to the orbits. Thus, there is a lot of uncertainty when comparing the evolution of semi-major axis of the orbit and the eccentricity of the candidates (Egal et al., 2017; Vida et al., 2018).
3 Results
3.1 Showers identification and validation
16 meteors in BRAMON’s database were found that could be associated with the asteroid 2019 OK. The mean orbital elements of the cluster were compared with the list of all meteor showers in the IAU Meteor Data Center. The comparison was made using the discrimination criteria DD, DSH and DH, using thresholds of 0.09, 0.15 and 0.15, respectively. No meteor showers in the catalog have been associated with the cluster and this group of meteors has been identified as a new annual meteor shower. Following the naming rules for meteor showers (Jenniskens, 2007; 2008) the shower was named 17 Capricornids, receiving from the IAU MDC the number 1042 and code SCP. The orbital data of the SCP shower, as well as the orbital data of asteroid 2019 OK can be seen in Table 1 and Table 2.
During the search, 20 meteors were found in the BRAMON’s database that could be associated with asteroid 2017 NT5. The same procedure was adopted for this second cluster. The mean orbital elements were compared with the list of all meteor showers of the IAU Meteor Data Center, using the same DD, DSH and DH thresholds. No meteor showers in the catalog have been associated with the cluster, therefore it was concluded that this group of meteors is part of a new annual meteor shower. Once again, following naming rules, the group was named omega Sagittariids, receiving from the IAU MDC the number 1043 and code OSG. The orbital elements data for the shower OSG as well as the orbital data for the asteroid 2017 NT5 can be seen in Table 3 and Table 4.
Table 1 – Orbit data for the meteors associated with the SCP (#1042) shower.
| Meteor | λ☉ (°) | αg (°) | δg (°) | vg (km/s) | a (A.U.) | e | q (A.U.) | ω (°) | Ω (°) | i (°) | DD |
| 20150716_044925 | 113.15 | 305.3 | –20.3 | 25.5 | 2.01 | 0.785 | 0.431 | 107.98 | 293.15 | 0.34 | 0.048 |
| 20160705_052435 | 103.39 | 293.7 | –22.4 | 23.5 | 1.84 | 0.738 | 0.480 | 104.04 | 283.38 | 0.19 | 0.091 |
| 20160716_034824 | 113.82 | 302.1 | –22.8 | 24.9 | 2.37 | 0.794 | 0.487 | 100.12 | 293.83 | 2.14 | 0.073 |
| 20160718_044750 | 115.77 | 308.9 | –21.9 | 25.8 | 2.11 | 0.794 | 0.433 | 107.12 | 295.78 | 2.50 | 0.043 |
| 20160801_224158 | 129.85 | 318.2 | –19.1 | 25.2 | 1.96 | 0.777 | 0.434 | 107.92 | 309.86 | 3.08 | 0.052 |
| 20160802_015622 | 129.98 | 323.9 | –23.7 | 25.6 | 1.97 | 0.711 | 0.432 | 108.03 | 309.99 | 9.18 | 0.076 |
| 20160811_010933 | 138.58 | 328.7 | –15.6 | 23.0 | 1.79 | 0.731 | 0.479 | 104.29 | 318.59 | 2.69 | 0.068 |
| 20170715_000726 | 112.48 | 300.6 | –21.6 | 25.3 | 2.01 | 0.780 | 0.439 | 107.10 | 292.48 | 1.32 | 0.045 |
| 20170715_075327 | 112.78 | 310.6 | –24.7 | 21.9 | 1.37 | 0.677 | 0.440 | 113.74 | 292.79 | 3.68 | 0.060 |
| 20170728_021802 | 124.98 | 315.6 | –19.5 | 26.8 | 2.40 | 0.823 | 0.422 | 107.01 | 304.98 | 2.33 | 0.060 |
| 20170807_070646 | 134.74 | 328.0 | –17.2 | 25.6 | 2.11 | 0.793 | 0.434 | 106.54 | 314.74 | 2.36 | 0.068 |
| 20180718_071555 | 115.38 | 307.2 | –20.8 | 24.7 | 2.05 | 0.775 | 0.460 | 104.58 | 295.41 | 0.83 | 0.038 |
| 20190811_051129 | 138.01 | 329.4 | –17.8 | 25.6 | 2.52 | 0.813 | 0.470 | 101.25 | 318.01 | 4.19 | 0.064 |
| 20200707_063450 | 105.33 | 298.7 | –26.2 | 24.9 | 1.89 | 0.766 | 0.442 | 107.56 | 285.33 | 4.43 | 0.069 |
| 20200723_023833 | 120.44 | 307.7 | –20.1 | 24.1 | 2.19 | 0.774 | 0.495 | 100.08 | 300.46 | 0.90 | 0.052 |
| 20200727_040356 | 124.31 | 315.9 | –18.6 | 26.5 | 2.32 | 0.816 | 0.425 | 106.98 | 304.33 | 1.29 | 0.055 |
| Means | 120.82 | 312.2 | –20.8 | 24.9 | – | 0.772 | 0.450 | 105.90 | 300.82 | 2.59 | – |
| Medians | 118.11 | 309.8 | –20.6 | 25.21 | – | 0.779 | 0.440 | 106.99 | 298.12 | 2.34 | – |
| 2019 OK | – | – | – | – | 1.87 | 0.757 | 0.453 | 106.09 | 302.04 | 2.09 | – |
Table 2 – Sun-centered radiants for the meteors associated with the SCP (#1042) shower.
| NumCur | CatCod | MetCod | λ☉ (°) | λg – λ☉ (°) | βg (°) | vg (km/s) | No | Code |
| 1 | BR | 20150716_044925 | 113.158 | 189.6 | –0.8 | 25.5 | 1042 | SCP |
| 2 | BR | 20160705_052435 | 103.399 | 188.4 | –0.8 | 23.5 | 1042 | SCP |
| 3 | BR | 20160716_034824 | 113.825 | 185.5 | –2.6 | 24.9 | 1042 | SCP |
| 4 | BR | 20160718_044750 | 115.772 | 189.9 | –3.1 | 25.8 | 1042 | SCP |
| 5 | BR | 20160801_224158 | 129.858 | 185.0 | –2.9 | 25.2 | 1042 | SCP |
| 6 | BR | 20160802_015622 | 129.988 | 188.5 | –8.9 | 25.6 | 1042 | SCP |
| 7 | BR | 20160811_010933 | 138.582 | 186.9 | –2.8 | 23.1 | 1042 | SCP |
| 8 | BR | 20170715_000726 | 112.480 | 185.8 | –1.1 | 25.3 | 1042 | SCP |
| 9 | BR | 20170715_075327 | 112.789 | 193.7 | –6.2 | 21.9 | 1042 | SCP |
| 10 | BR | 20170728_021802 | 124.980 | 187.4 | –2.6 | 26.8 | 1042 | SCP |
| 11 | BR | 20170807_070646 | 134.740 | 189.6 | –4.1 | 25.6 | 1042 | SCP |
| 12 | BR | 20180718_071555 | 115.386 | 189.1 | –1.7 | 24.8 | 1042 | SCP |
| 13 | BR | 20190811_051129 | 138.010 | 187.4 | –5.1 | 25.6 | 1042 | SCP |
| 14 | BR | 20200707_063450 | 105.333 | 190.3 | –5.3 | 24.9 | 1042 | SCP |
| 15 | BR | 20200723_023833 | 120.440 | 184.6 | –1.1 | 24.1 | 1042 | SCP |
| 16 | BR | 20200727_040356 | 124.318 | 188.6 | –1.8 | 26.5 | 1042 | SCP |
Table 3 – Orbit data of the meteors associated with the OSG (#1043) shower.
| Meteor | λ☉ (°) | αg (°) | δg (°) | vg (km/s) | a (A.U.) | e | q (A.U.) | ω (°) | Ω (°) | i (°) | DD |
| 20140803_060048 | 130.64 | 315.8 | –23.3 | 20.3 | 2.15 | 0.710 | 0.621 | 86.20 | 310.65 | 3.17 | 0.068 |
| 20150702_231514 | 100.54 | 278.0 | –25.5 | 19.7 | 1.80 | 0.660 | 0.608 | 90.58 | 280.55 | 1.68 | 0.064 |
| 20160706_053735 | 104.36 | 291.9 | –29.6 | 20.7 | 1.80 | 0.680 | 0.575 | 94.18 | 284.36 | 5.15 | 0.067 |
| 20160707_064949 | 105.36 | 293.1 | –27.4 | 19.0 | 1.62 | 0.630 | 0.599 | 93.56 | 285.36 | 2.77 | 0.061 |
| 20160812_011335 | 139.54 | 318.2 | –24.8 | 18.2 | 1.98 | 0.660 | 0.676 | 80.63 | 319.55 | 4.78 | 0.070 |
| 20170703_013859 | 101.10 | 280.0 | –24.8 | 19.3 | 1.84 | 0.660 | 0.626 | 88.16 | 281.11 | 1.27 | 0.064 |
| 20170703_035627 | 101.19 | 282.2 | –35.4 | 20.4 | 2.12 | 0.700 | 0.638 | 84.54 | 281.19 | 8.35 | 0.070 |
| 20170715_002439 | 112.49 | 288.1 | –23.6 | 20.4 | 2.38 | 0.730 | 0.643 | 82.58 | 292.49 | 0.96 | 0.060 |
| 20170718_034313 | 115.49 | 295.8 | –28.8 | 19.6 | 2.16 | 0.700 | 0.656 | 82.11 | 295.48 | 4.51 | 0.026 |
| 20170723_013538 | 120.17 | 297.9 | –33.9 | 19.0 | 2.22 | 0.690 | 0.693 | 77.36 | 300.17 | 7.86 | 0.038 |
| 20170723_040407 | 120.27 | 302.6 | –32.4 | 21.5 | 2.68 | 0.760 | 0.638 | 81.94 | 300.27 | 8.06 | 0.062 |
| 20170725_043636 | 122.21 | 307.8 | –23.8 | 20.1 | 1.84 | 0.680 | 0.594 | 91.71 | 302.21 | 2.58 | 0.068 |
| 20170726_021756 | 123.07 | 301.0 | –27.0 | 19.4 | 2.29 | 0.710 | 0.673 | 79.34 | 303.07 | 4.20 | 0.029 |
| 20180716_003506 | 113.21 | 285.8 | –24.2 | 18.2 | 2.14 | 0.670 | 0.698 | 77.17 | 293.23 | 0.78 | 0.066 |
| 20180730_001439 | 126.56 | 302.2 | –18.9 | 19.3 | 2.22 | 0.700 | 0.663 | 80.91 | 306.77 | 0.03 | 0.049 |
| 20200719_234531 | 117.46 | 292.4 | –24.3 | 19.2 | 2.10 | 0.690 | 0.659 | 82.17 | 297.47 | 1.63 | 0.032 |
| 20200721_011019 | 118.47 | 294.7 | –24.5 | 20.1 | 2.44 | 0.730 | 0.660 | 80.29 | 298.48 | 2.07 | 0.049 |
| 20200721_054110 | 118.65 | 306.4 | –34.2 | 19.6 | 1.89 | 0.660 | 0.635 | 86.63 | 298.65 | 8.97 | 0.028 |
| 20200722_063521 | 119.64 | 304.4 | –22.5 | 19.2 | 1.85 | 0.660 | 0.630 | 88.04 | 299.67 | 0.49 | 0.048 |
| 20200806_013302 | 133.78 | 307.8 | –25.8 | 18.7 | 2.61 | 0.730 | 0.718 | 72.19 | 313.78 | 3.92 | 0.062 |
| Means | 117.21 | 297.3 | –26.7 | 19.6 | – | 0.690 | 0.645 | 84.01 | 297.23 | 3.66 | – |
| Medians | 118.56 | 296.8 | –25.2 | 19.5 | – | 0.690 | 0.641 | 82.37 | 298.57 | 2.97 | – |
| 2017 NT5 | – | – | – | – | 1.87 | 0.676 | 0.605 | 88.94 | 293.57 | 6.04 | – |
Table 4 – Sun-centered radiants for the meteors associated with the OSG (#1043) shower.
| NumCur | CatCod | MetCod | λ☉ (°) | λg – λ☉ (°) | βg (°) | vg (km/s) | No | Code |
| 1 | BRAM | 20140803_060048 | 130.649 | 180.8 | –6.2 | 20.3 | 1043 | OSG |
| 2 | BRAM | 20150702_231514 | 100.541 | 176.7 | –2.3 | 19.7 | 1043 | OSG |
| 3 | BRAM | 20160706_053735 | 104.361 | 184.7 | –7.6 | 20.7 | 1043 | OSG |
| 4 | BRAM | 20160707_064949 | 105.363 | 185.1 | –5.6 | 19.0 | 1043 | OSG |
| 5 | BRAM | 20160812_011335 | 139.544 | 173.6 | –8.3 | 18.2 | 1043 | OSG |
| 6 | BRAM | 20170703_013859 | 101.102 | 177.9 | –1.6 | 19.3 | 1043 | OSG |
| 7 | BRAM | 20170703_035627 | 101.193 | 178.9 | –12.4 | 20.4 | 1043 | OSG |
| 8 | BRAM | 20170715_002439 | 112.491 | 174.0 | –1.2 | 20.4 | 1043 | OSG |
| 9 | BRAM | 20170718_034313 | 115.485 | 177.1 | –7.4 | 19.6 | 1043 | OSG |
| 10 | BRAM | 20170723_013538 | 120.174 | 173.3 | –12.6 | 19.0 | 1043 | OSG |
| 11 | BRAM | 20170723_040407 | 120.273 | 177.5 | –12.0 | 21.5 | 1043 | OSG |
| 12 | BRAM | 20170725_043636 | 122.205 | 182.1 | –4.7 | 20.1 | 1043 | OSG |
| 13 | BRAM | 20170726_021756 | 123.069 | 174.4 | –6.5 | 19.4 | 1043 | OSG |
| 14 | BRAM | 20180716_003506 | 113.212 | 171.2 | –1.5 | 18.2 | 1043 | OSG |
| 15 | BRAM | 20180730_001439 | 126.564 | 173.7 | +1.2 | 19.3 | 1043 | OSG |
| 16 | BRAM | 20200719_234531 | 117.461 | 172.9 | –2.4 | 19.2 | 1043 | OSG |
| 17 | BRAM | 20200721_011019 | 118.472 | 173.9 | –3.0 | 20.1 | 1043 | OSG |
| 18 | BRAM | 20200721_054110 | 118.651 | 181.8 | –14.5 | 19.6 | 1043 | OSG |
| 19 | BRAM | 20200722_063221 | 119.64 | 181.8 | –2.7 | 19.2 | 1043 | OSG |
| 20 | BRAM | 20200806_013302 | 133.782 | 169.9 | –6.7 | 18.7 | 1043 | OSG |
The asteroid 2019 OK was first detected on July 24, 2019, at distance of 0.01 AU (~1500000km) from Earth and had an apparent magnitude of 14.7, being observed in the constellation of Capricorn. With a MOID of 0.000381434 AU (NASA JPL) and an absolute magnitude (H) = 23.3 (MPO473725), it was classified as a NEO (Near Earth Object) of the Apollo class. The asteroid was initially detected by Cristóvão Jacques, Eduardo Pimentel and João Ribeiro at the SONEAR Observatory, located in Oliveira, State of Minas Gerais, Brazil.

Figure 1 – First image of Asteroid 2019 OK. Courtesy SONEAR Observatory.

Figure 2 – Flyby (top view) by asteroid 2019 OK.
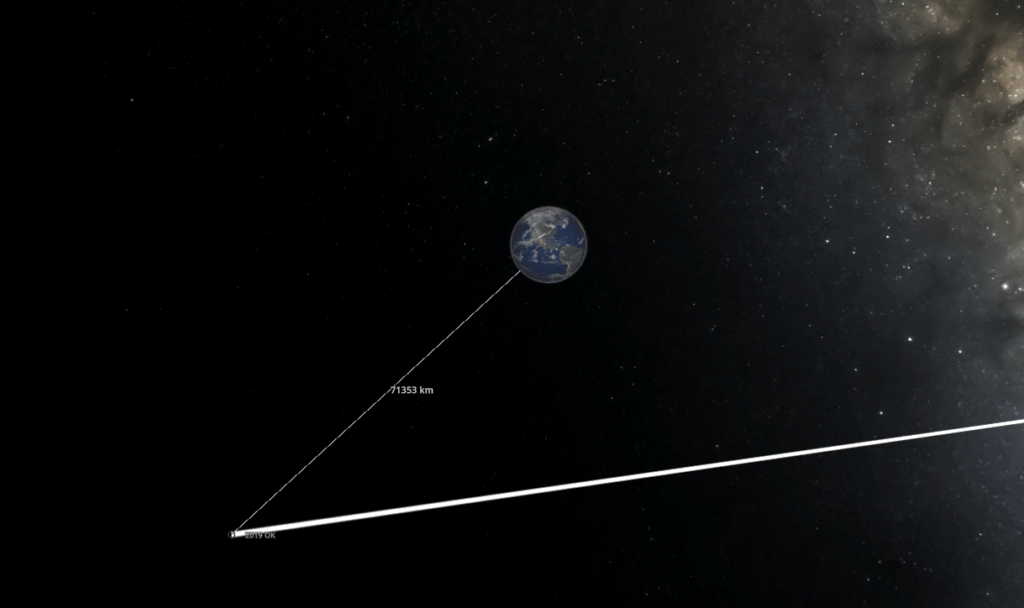
Figure 3 – Flyby (side view) by asteroid 2019 OK.
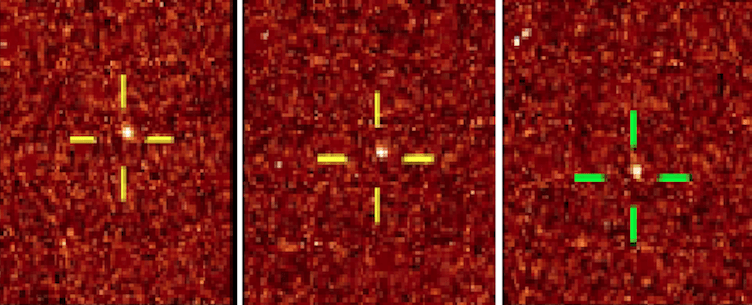
Figure 4 – First image of Asteroid 2017 NT5, Courtesy SONEAR Observatory.
The asteroid 2017 NT5 was first detected on July 12, 2017, by the SONEAR observatory. After more observations, the asteroid had its MOID calculated as 0.00257522 AU (~335381km) AU and an absolute magnitude (H) = 22.9 (MPO 416462), being classified as a NEO (Near Earth Object) of the Apollo class. Its flyby occurred on July 14, 2017 at 16h06mUT, when the asteroid reached a minimum distance of 0.002859082 AU (NASA JPL). It has an estimated size between 49 and 160m with an orbital period of 2.55 years (NASA JPL).
Using the orbit data from 2019 OK and 2017 NT5, the theoretical radiant (Neslušan et al., 1998) was calculated for possible meteor showers that could exist having these asteroids as parent bodies. The theoretical radiant calculated for asteroid 2019 OK was compared with the radiants observed for the meteors of SCP#1042 (Figure 5) listed in Table 1 and Table 2. The theoretical radiant calculated for the asteroid 2017 NT5 was compared with the radiants observed for the meteors of OSG#1043 (Figure 6) listed in Table 3 and Table 4. The results are presented in Table 5 and Table 6.
Table 5 – Observed radiant (SCP #1042) compared to the theoretical radiant (asteroid 2019 OK).
| Body | λ☉ (°) | αg (°) | δg (°) | vg (km/s) | a (A.U.) | e | q (A.U.) | ω (°) | Ω (°) | i (°) | D–Dis |
| 2019 OK | – | – | – | – | 1.87 | 0.757 | 0.453 | 106.09 | 302.04 | 2.09 | – |
| SCP #1042 | 120.81 | 312.2 | –20.8 | 24.9 | 2.06 | 0.772 | 0.451 | 105.90 | 300.82 | 2.60 | – |
| 2019 OK Fitted orbit | 121.60 | 312.1 | –20.2 | 24.3 | 1.87 | 0.757 | 0.454 | 106.50 | 301.60 | 2.10 | 0.000 |
Table 6 – Observed radiant (OSG #1043) compared to the theoretical radiant (asteroid 2017 NT5).
| Body | λ☉ (°) | αg (°) | δg (°) | vg (km/s) | a (A.U.) | e | q (A.U.) | ω (°) | Ω (°) | i (°) | D–Dis |
| 2017 NT5 | – | – | – | – | 1.87 | 0.676 | 0.605 | 88.94 | 293.57 | 6.04 | – |
| OSG #1043 | 117.21 | 297.3 | –26.7 | 19.6 | 1.94 | 0.645 | 0.690 | 84.02 | 297.23 | 3.66 | – |
| 2017 NT5 Fitted orbit | 112.40 | 295.6 | –30.4 | 20.2 | 0.676 | 0.605 | 90.20 | 292.40 | 6.00 | 0.002 |
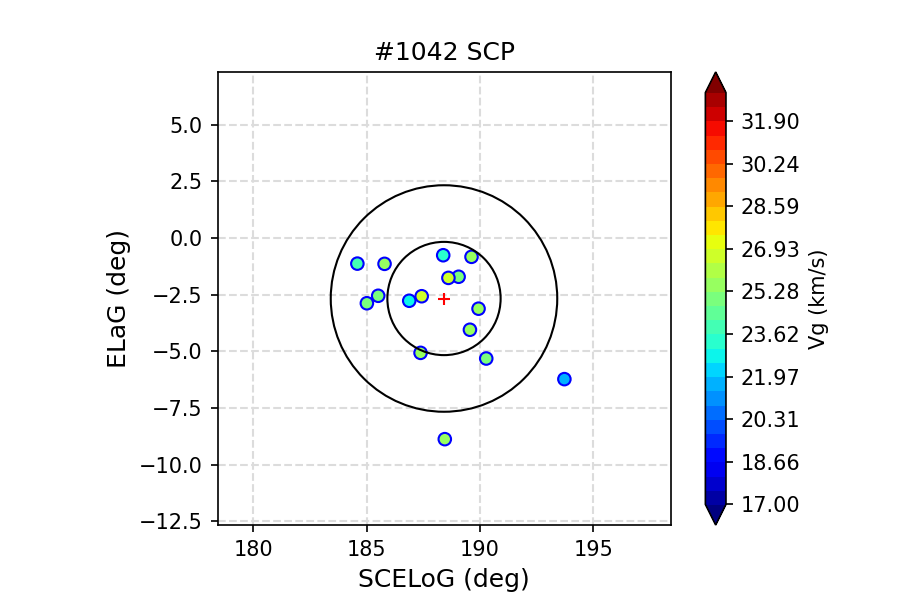
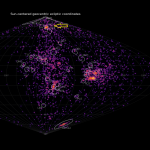
Figure 5 – The SCP radiants in Sun-centered coordinates. The concentric circles represent distances of 5° and 10° from the mean geocentric radiant.
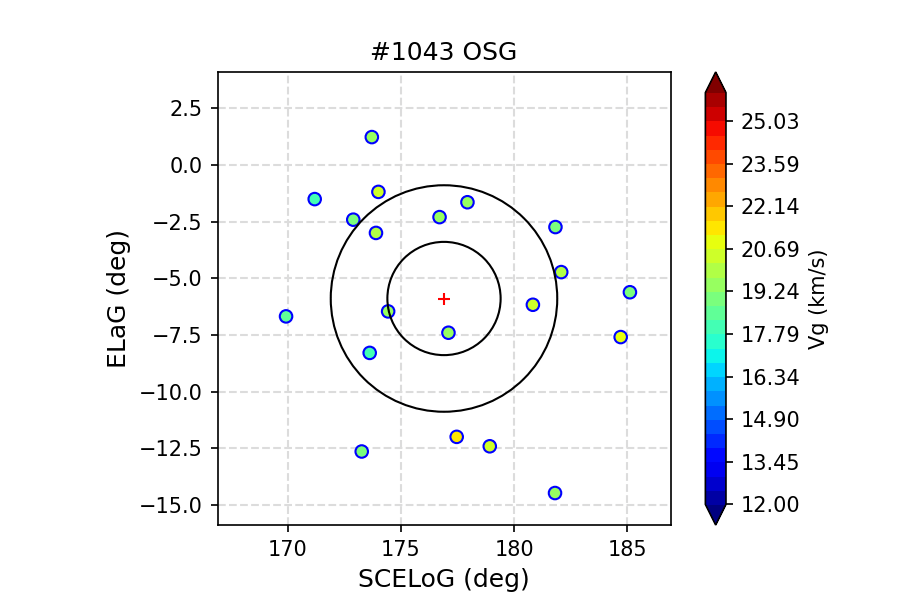
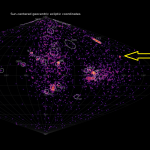
Figure 6 – The OSG radiants in Sun-centered coordinates. The concentric circles represent distances of 5° and 10° from the mean geocentric radiant.
It is often difficult to determine the limits of comparison between the original orbits of asteroids and the adjusted orbits (Neslušan et al., 1998). Depending on the method used for adjusting such orbits one can eventually discard good candidates for parent bodies. The DSH-criterion appears as a parameter for measuring the uncertainty about the confirmation of a theoretical radiant (Svoren et al., 1993; 1994; Neslušan et al., 1998). The suggestion to use DSH as a discriminant (Neslušan et al., 1998) is not a definitive measure, but only a form of quality assessment of the approximation between the original orbit of the tested asteroid and its adjusted orbit for an intersection with the Earth orbit. Thus, D-Dis and DSH have the same concept: both measure dissimilarity between orbits. It is possible to accept the similarity between the mean orbit of the shower and the parent body if D-Dis < 0.07 and, if the distance between the two radiants does not exceed 5º, it is possible to accept a D-Dis value of maximum 0.1 (Neslušan et al., 1998). For the asteroid 2019 OK and the SCP meteor shower, a distance of 0.6º was found between the theoretical radiant and the observed radiant. For asteroid 2017 NT5 and the OSG meteor shower, the distance between the theoretical and observed radiant is 3.96º.
Another criterion used to associate meteors with parent bodies also includes the determination of the theoretical radiant (Neslušan et al., 1998) and has the following acceptable tolerances: Δλ☉ < 8º, Δα < 8º, Δδ < 8º and ΔΩ < 6 º (Guennoun et al., 2019). Both the SCP and OSG showers meet the criteria suggested by Guennoun et al. (2019) for their association with asteroid 2019 OK and 2017 NT5 respectively.
3.2 Backward Integration
The interpretation of the charts after backward integration showed that the evolution of the mean orbital elements of the meteor clusters (Williams and Jones, 2007) and their candidate asteroidal parent bodies had similar behavior within the time interval studied. The thresholds used were: DSH = 0.15; DD = 0.07 and DH = 0.15.

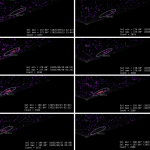
Figure 7 – The orbit (green line) and the position of the asteroid 2019 OK at the close approach in 2019 (Top view) and the mean orbit of the SCP meteor shower in blue line.
Figure 7 shows the position of the planets of the inner solar system at the time of the close approach of the asteroid 2019 OK, as well as the orbits of the asteroid itself (green line) and the mean orbit of the SCP meteors (blue line).
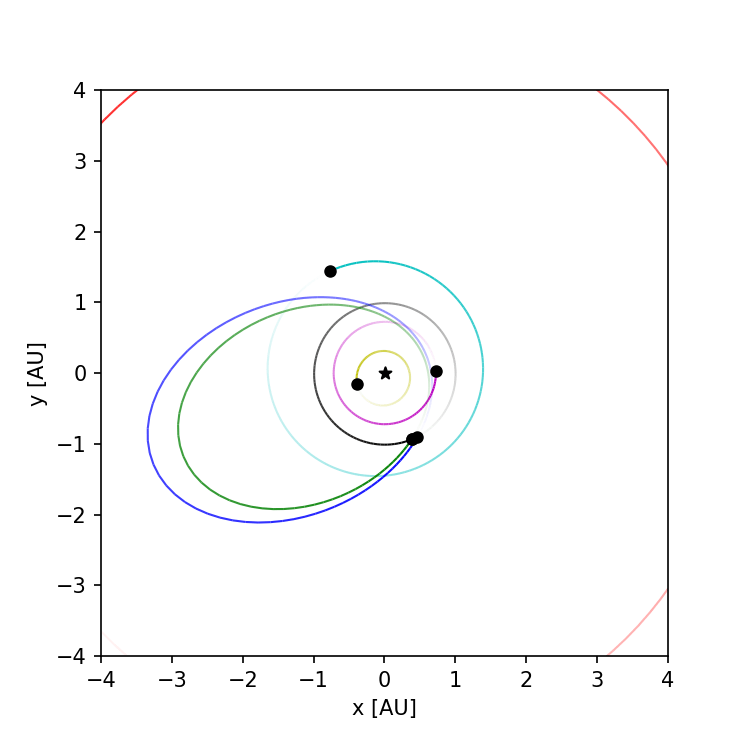
Figure 8 – The orbit (green line) and the position of asteroid 2017 NT5 at the close approach in 2017 (Top view) and the mean orbit of the OSG meteor shower in blue line.
The same workflow was applied in the research for shower #1043 OSG and its parent body candidate asteroid 2017 NT5. Figure 8 shows the position of the planets of the inner solar system at the time of the close approach of the asteroid 2017 NT5, as well as the orbits of the asteroid itself (green line) and the mean orbit of the OSG meteors.
3.3. New Meteor Showers Elements
SCP (#1042)
The meteor shower SCP (#1042) can be found in the IAU Meteor Data Center (MDC) section “List of all showers” (Jenniskens et al., 2020). The meteors radiate from a mean geocentric radiant at RA = 312.2º and Dec.= –20. 8º, with a geocentric velocity of 24.93 km/s. The meteor shower SCP (#1042) has a peak at λ☉ = 120.82° on 2020 July 23 at 12h UT (equinox J2000.0). The group of 16 meteors has the following mean orbital elements:
- a ~ 2.06 AU
- q = 0.450 AU
- e = 0.772
- i = 2.6°
- ω = 105.9°
- Ω = 300.8°
After comparing the evolution of the orbital elements of the asteroid 2019 OK and the meteor shower SCP (#1042) over past time, it appeared that they have similarity in the last 5000 years (Figures 9 to 14). The comparison between the theorical radiant position for the asteroid and the radiant observed for the meteor shower meets the similarity parameters (Neslušan et al., 1998; Guennoun et al., 2019). Thus, the asteroid 2019 OK is selected as the probable parent body of meteor shower SCP (#1042).
OSG (#1043)
The meteor shower OSG (#1043) can be found in the IAU Meteor Data Center (MDC) section “List of all showers” (Jenniskens et al., 2020). The meteors radiated from a mean geocentric radiant at RA = 297.3° and Dec. = –26.7º, with a geocentric velocity of 19.59 km/s. The meteor shower OSG (#1043) has a peak at λ☉ = 117.21° on 2020 July 19 at 18h UT (equinox J2000.0). The 20 meteors give the following mean orbital elements:
- a ~ 2.11 AU
- q = 0.6451 AU
- e = 0.69
- i = 3.7°
- ω = 84.01°
- Ω = 297.23°
After comparing the evolution of the orbital elements of the asteroid 2017 NT5 and the meteor shower OSG (#1043), it appeared that they keep similar in the last 5000 years (Figures 15 to 20). Also, the comparison between the theoretical radiant position for the asteroid and the radiant observed for the meteor shower meets the similarity parameters (Neslušan et al., 1998; Guennoun et al., 2019). Thus, the asteroid 2017 NT5 is considered to be the probable parent body of the meteor shower OSG (#1043).
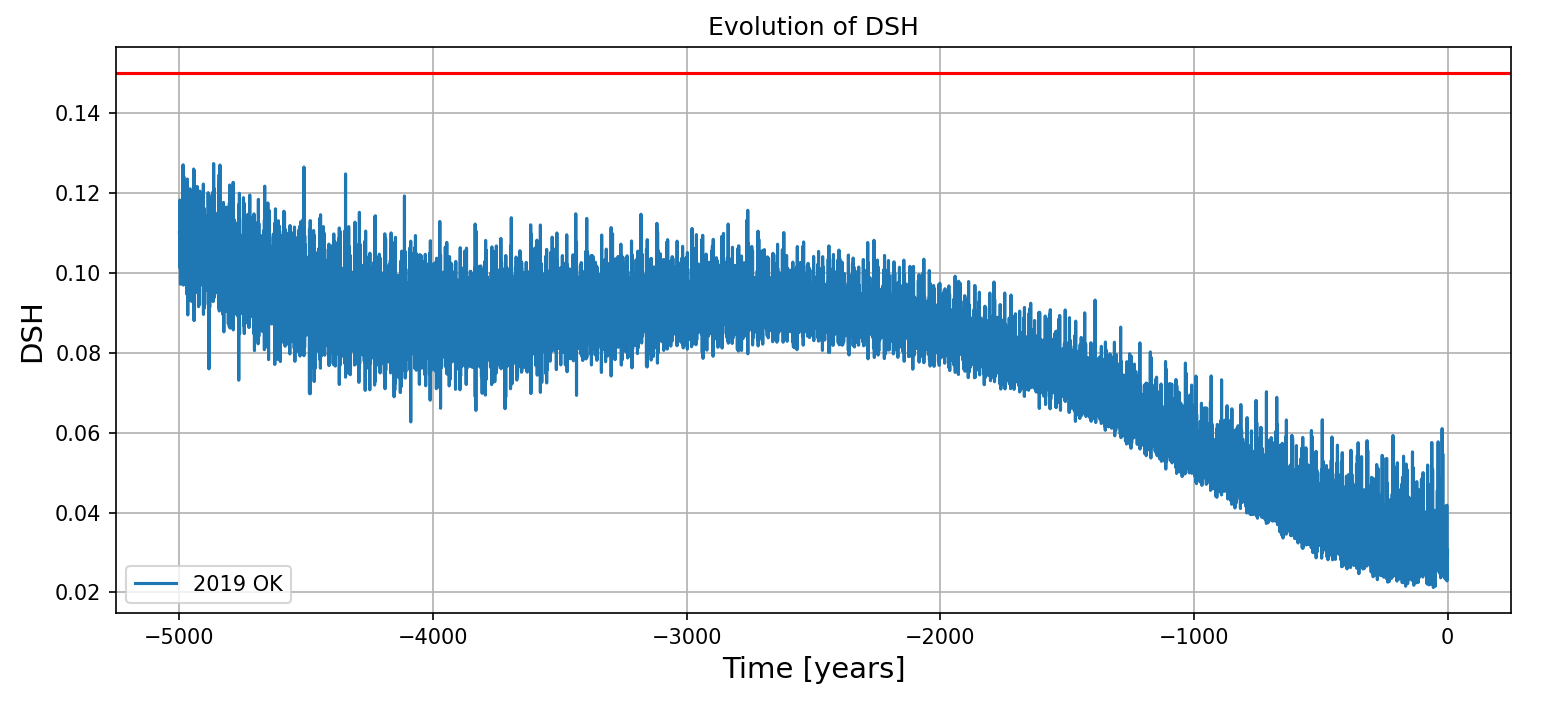
Figure 9 – DSH values for the orbit of the asteroid 2019 OK compared to the mean orbit of SCP meteors in the last 5000 years.
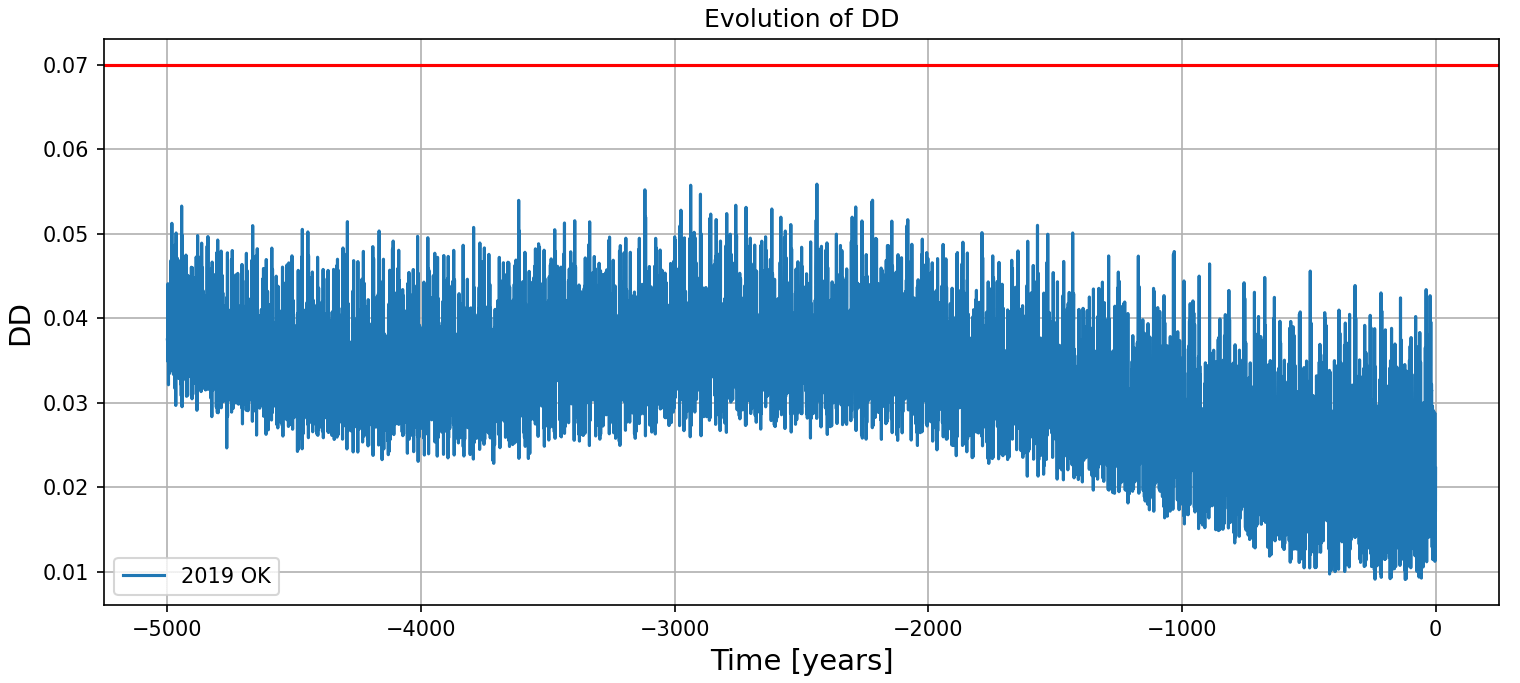
Figure 10 – DD values for the orbit of the asteroid 2019 OK compared to the mean orbit of SCP meteors in the last 5000 years.
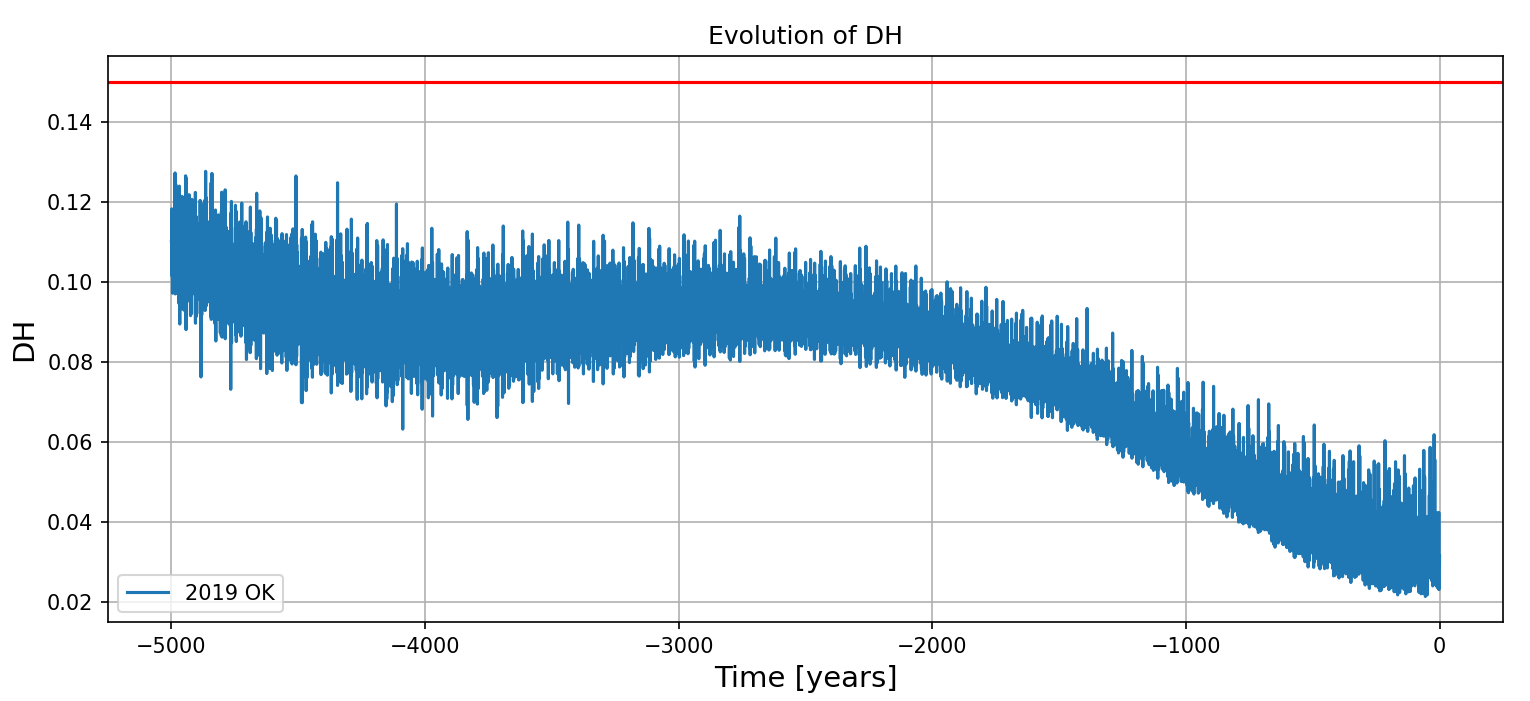
Figure 11 – DH values for the orbit of the asteroid 2019 OK compared to the mean orbit of SCP meteors in the last 5000 years.
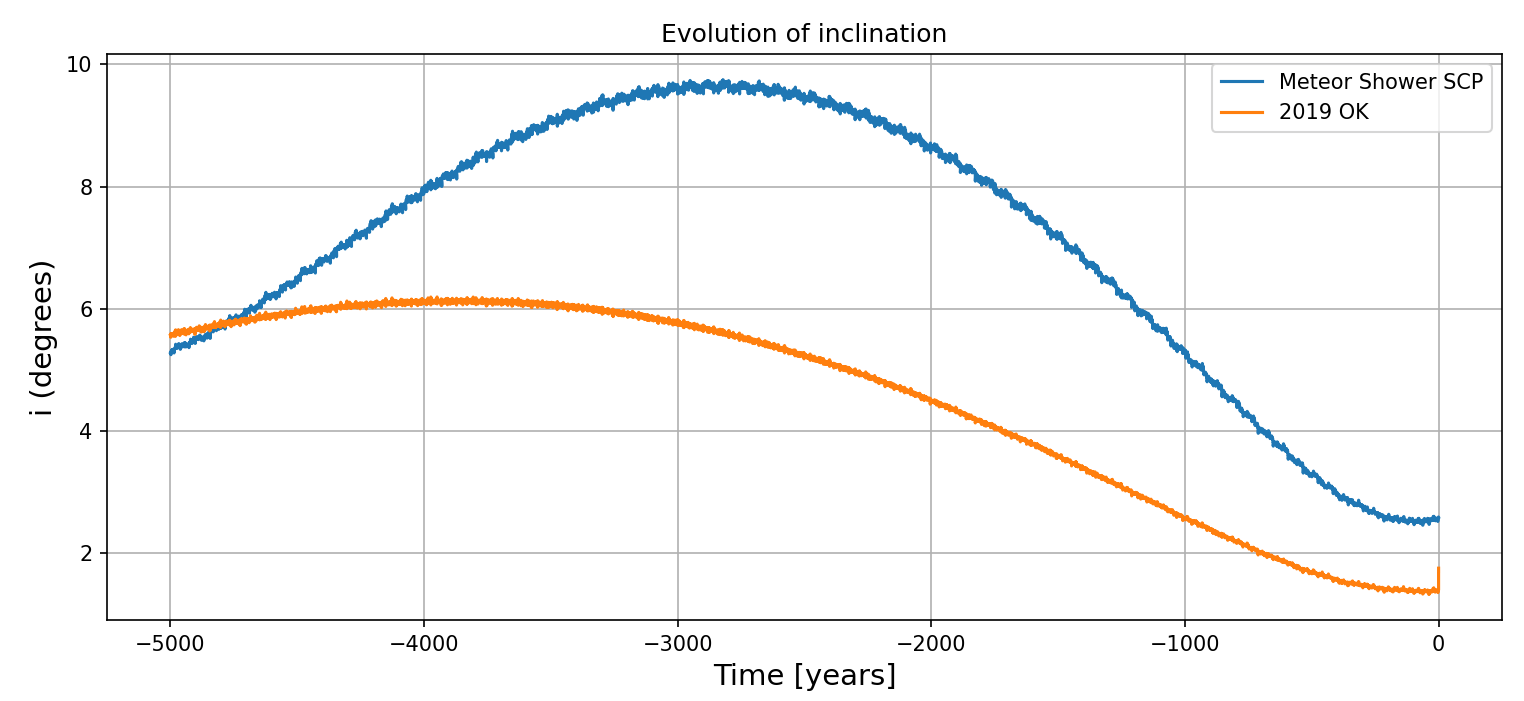
Figure 12 – Evolution of the orbit inclination i of asteroid 2019 OK and the median orbit of SCP meteors in the last 5000 years.
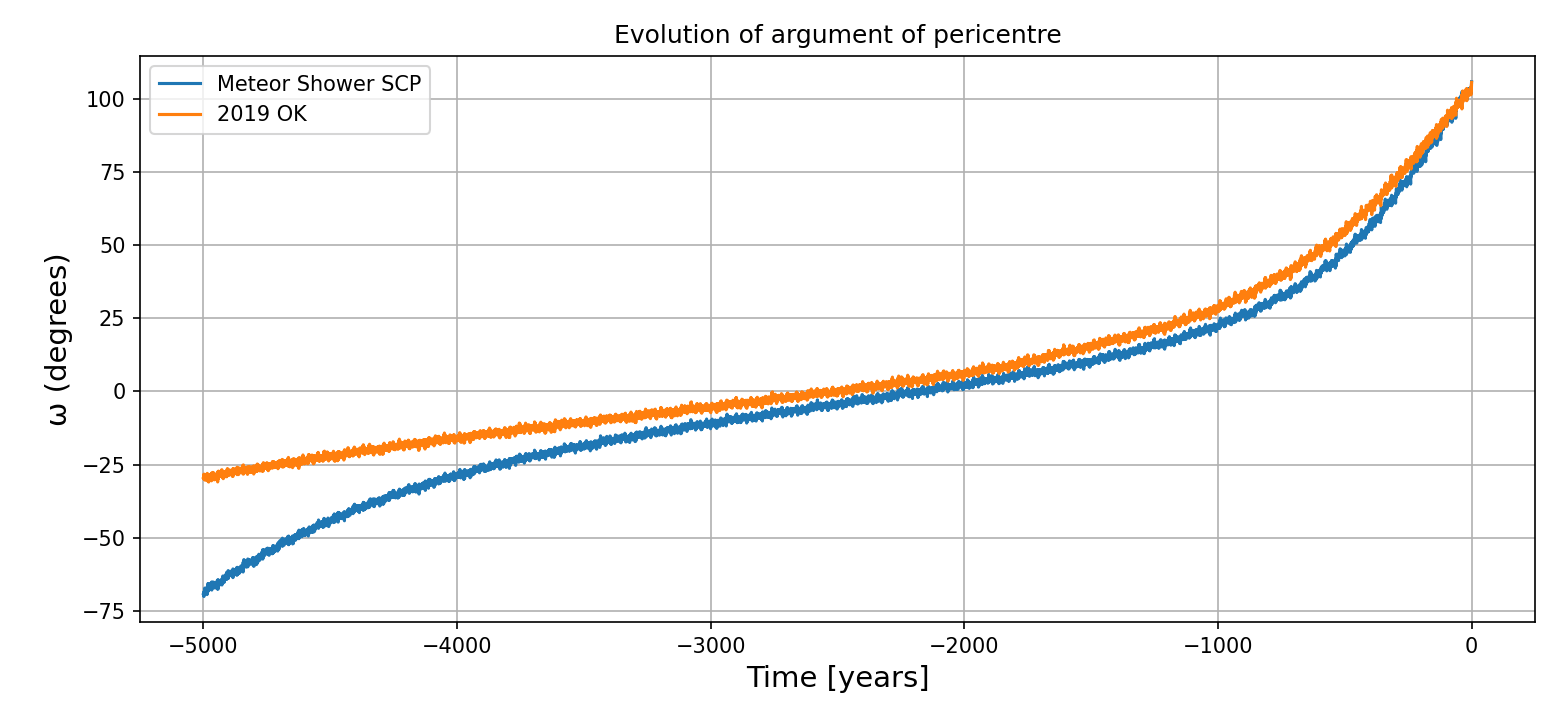
Figure 13 – Evolution of the argument of perihelion ω of asteroid 2019 OK and the mean orbit of SCP meteors in the last 5000 years.
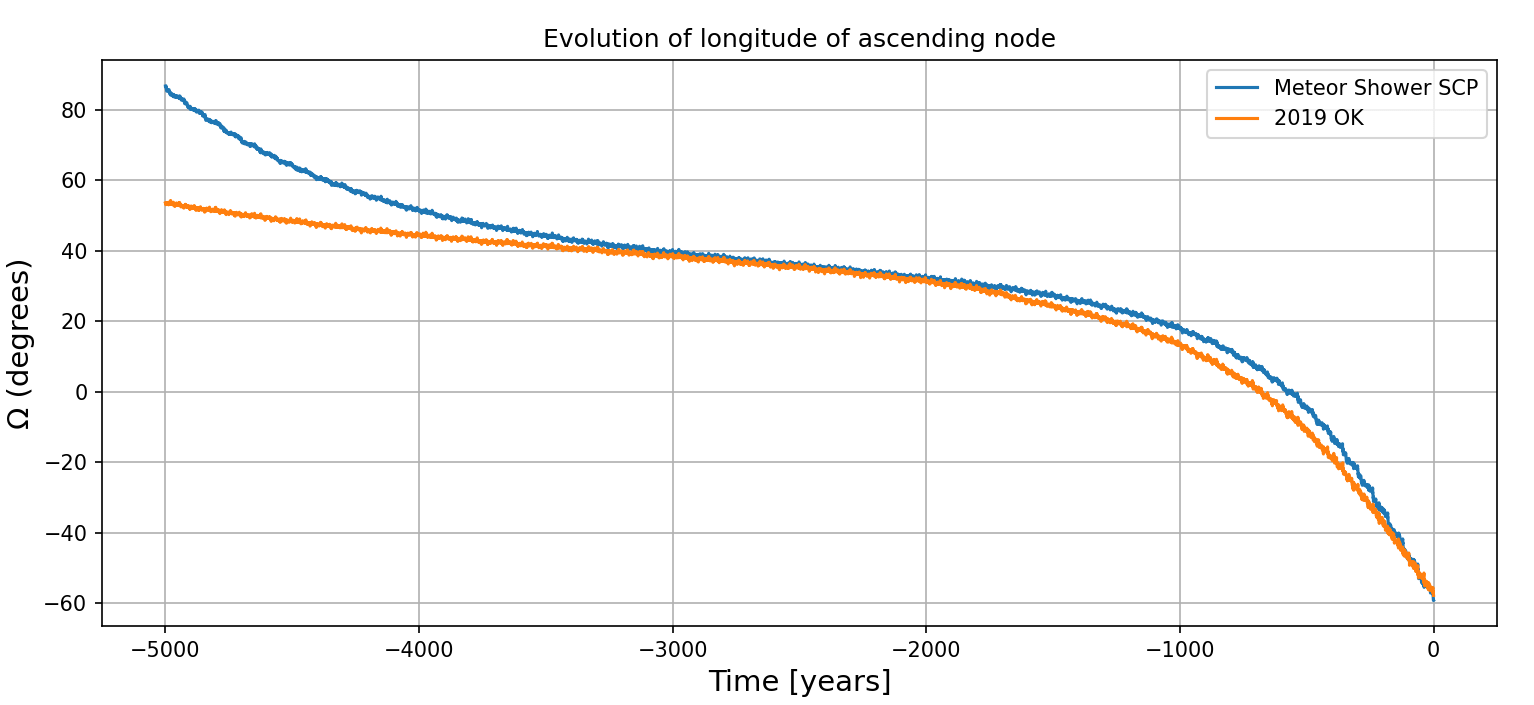
Figure 14 – Evolution of longitude of the ascending node Ω of asteroid 2019 OK and the mean orbit of SCP meteors in the last 5000 years.
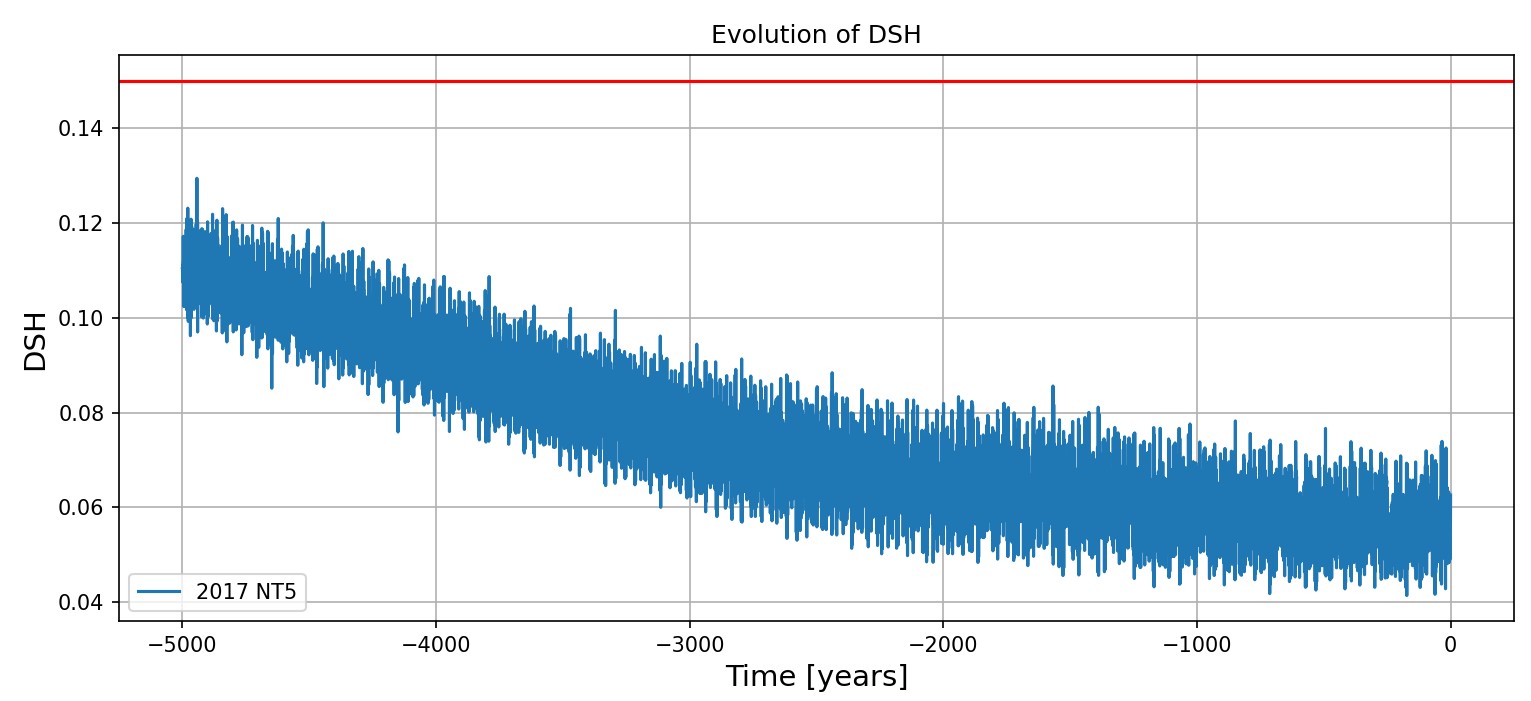
Figure 15 – DSH values for the orbit of the asteroid 2017 NT5 compared to the mean orbit of OSG meteors in the last 5000 years.
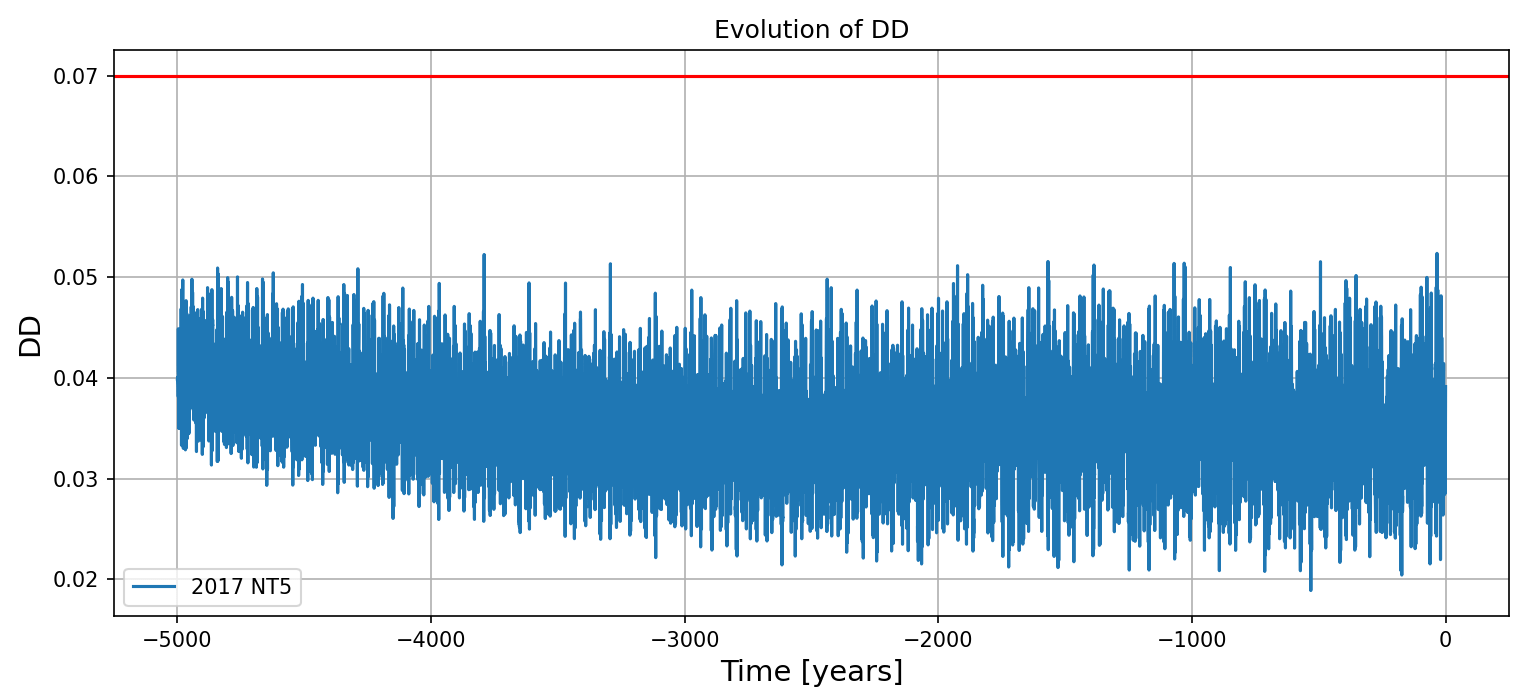
Figure 16 – DD values for the orbit of the asteroid 2017 NT5 compared to the mean orbit of OSG meteors in the last 5000 years.
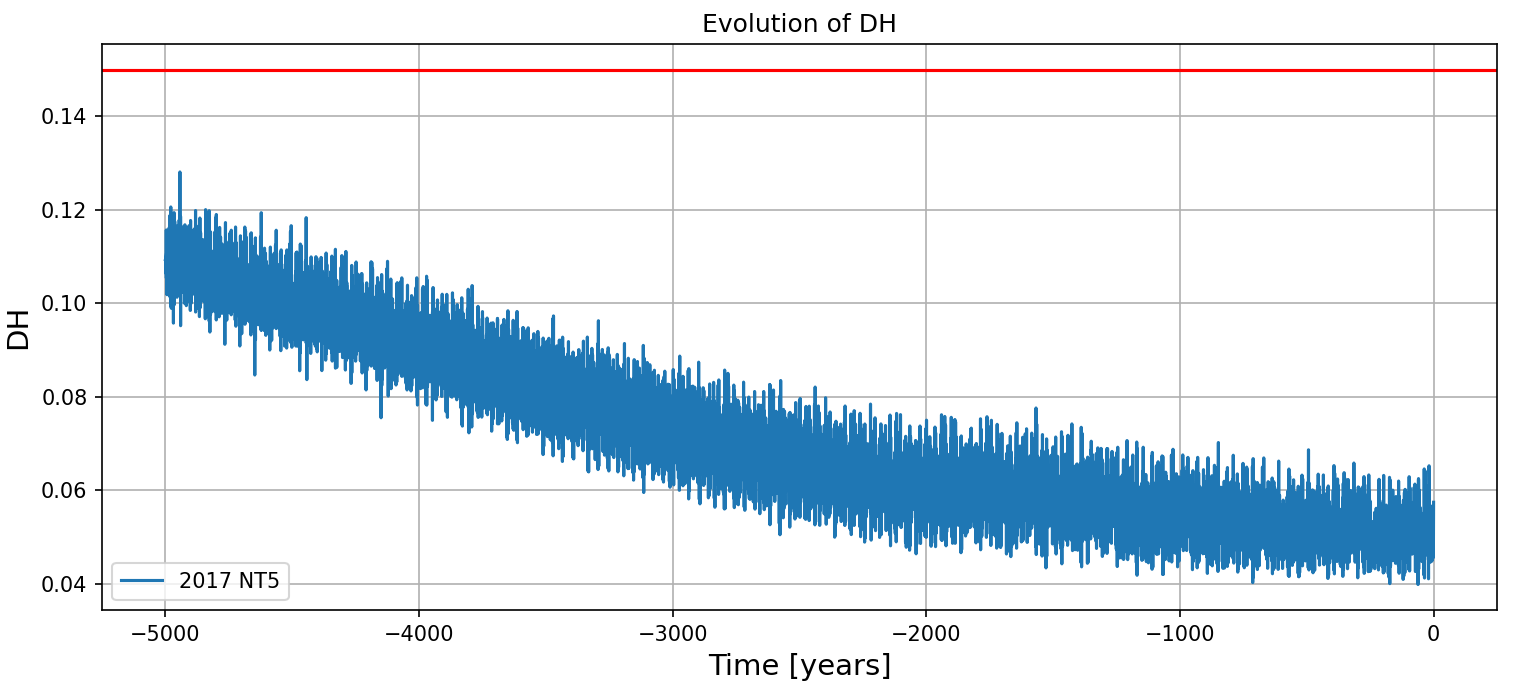
Figure 17 – DH values for the orbit of the asteroid 2017 NT5 compared to the mean orbit of OSG meteors in the last 5000 years.
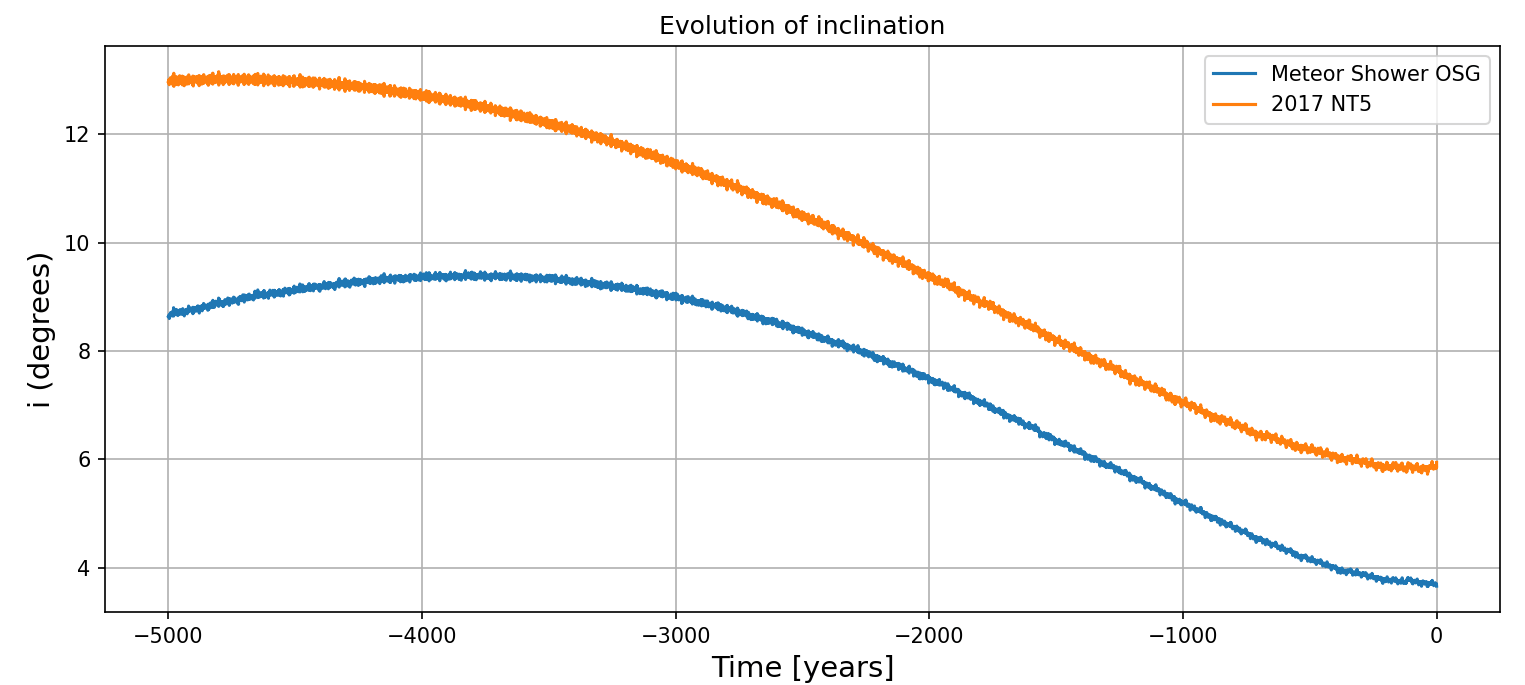
Figure 18 – Evolution of the orbit inclination i of asteroid 2017 NT5 and the mean orbit of OSG meteors in the last 5000 years.
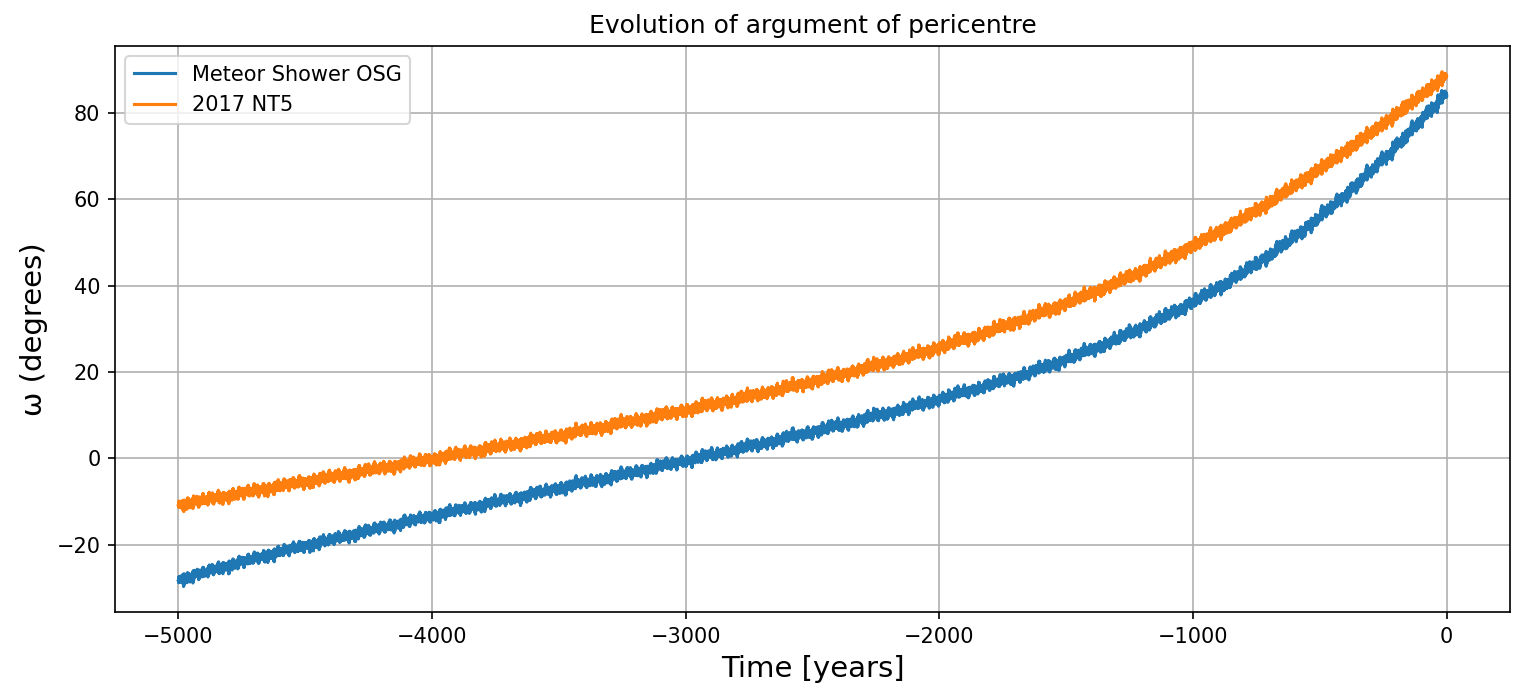
Figure 19 – Evolution of the argument of perihelion ω of the orbit of the asteroid 2017 NT5 and the mean orbit of OSG meteors in the last 5000 years.
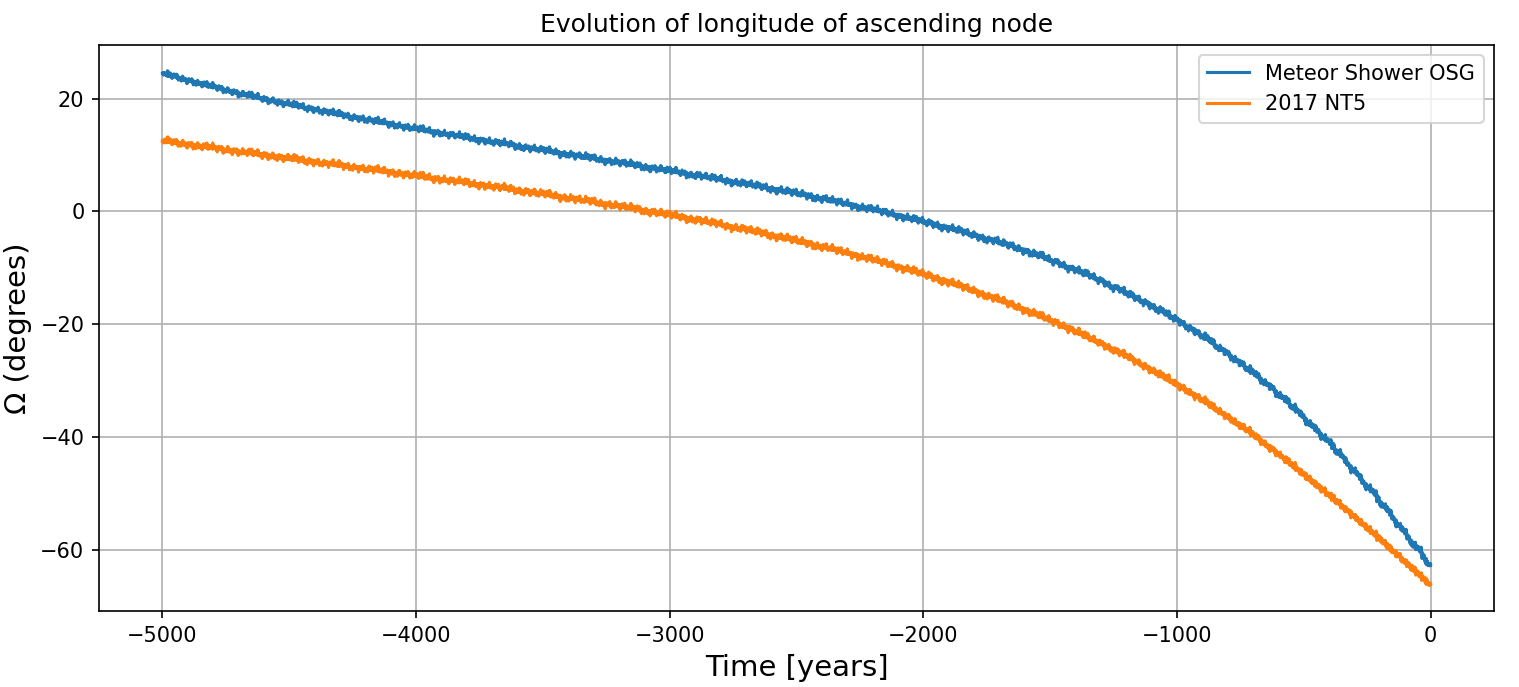
Figure 20 – Evolution of the longitude of the ascending node Ω of the orbit of the asteroid 2017 NT5 and the mean orbit of OSG meteors in the last 5000 years.
4 Conclusion
Using the different dissimilarity criteria and adopting very strict threshold values, it was possible to find in the BRAMON’s database two distinct groups of meteors that correspond to two new meteor showers for the southern hemisphere: the SCP (#1042) and OSG (#1043) showers. The mean orbital elements of these showers were obtained and compared to showers already accepted to guarantee their uniqueness. Thus, these two showers became the third and fourth showers from the southern hemisphere established by BRAMON with all or almost all of the meteors being found in its database. Finally, using a strict dissimilarity criterion and the backward integration of the showers and asteroids, it was possible to pinpoint the most probable parent body of the particles responsible for the meteor showers. The asteroids 2019 OK and 2017 NT5 show great similarity with the showers and were discovered by the SONEAR observatory, showing a good interaction of the research developed by the two Brazilian institutions.
Acknowledgment
We would like to thank all station operators at BRAMON engaged in producing data for meteor studies. A special thanks to the operators who contributed with the orbits used in this study:
Stations and operators who contributed with data for the discovery of shower SCP (#1042):
- ADJ1: Alfredo Dal’Ava Júnior;
- ARL1, ARL 2: Andrei Lima;
- CFJ3, CFJ4: Heller & Jung Observatory (Carlos Jung);
- ISL: Zaac Leite;
- JPZ2: Marcelo Zurita;
- LSA1: João Amâncio;
- OCA3: Leonardo Amaral;
- RCP2: Renato Poltronieri;
- ROO: Vandson Guedes;
- SMZ4, SMZ6: Sergio Mazzi;
- SON: Sonear Observatory (Cristovão Jacques);
- VRG: Wellington Albertini;
- UNB1: Ubiratan da Nóbrega Borges.
Stations and operators who contributed with data for the discovery of OSG (#1043) shower:
- AAC: Alcione Caetano;
- ADJ1: Alfredo Dal’Ava Júnior;
- BFB: Bruno Bonicontro;
- CFJ4, CFJ6, CFJ8: Heller & Jung Observatory (Carlos Jung);
- DLM: Daniel Leal Mateus;
- GZT: Gabriel Zaparolli;
- JJS: Jocimar Justino de Souza;
- JZL: Juliano Cezar Vieira Zaikievicz;
- LSA: João Amancio;
- OCA3: Leonardo Amaral;
- RCP2: Renato Poltronieri;
- RSC: Rafael Compassi;
- SMZ6: Sergio Mazzi;
- SON: SONEAR Observatory (Cristovão Jacques);
- WSR: William Siqueira.
Also, the authors would like to thank Lubos Neslušan for providing the algorithm for calculating the theoretical radiant (Neslušan et al., 1998) and the Western Meteor Physics Group for the dissimilarity criterion algorithms implementation (Vida., 2019).
References
Amaral L. S., Trindade, L. S., Bella C. A. P. B., Zurita M. L. P. V., Poltronieri R. C., Silva G. G., Faria C. J. L., Jung C. F., and Koukal J. A. (2018). “Brazilian Meteor Observation Network: History of creation and first developments”. In Gyssens M. and Rault J.-L., editors, Proceedings of the International Meteor Conference, Petnica, Serbia, 21–24 September, 2017. IMO. pages 171–175.
Bhatnagar K. B., Saha L. M. (1993). “N-Body problem”. Astronomical Society of India, Bulletin, 21, 1–25.
Brown P., Jones J. (1998). “Simulation of the Formation and Evolution of the Perseid Meteoroid Stream”. Icarus, 133, 36–68.
Chambers J. E. (1999). “A Hybrid Symplectic Integrator that Permits Close Encounters Between Massive Bodies”. Monthly Notices of the Royal Astronomical Society, 304, 793–799.
Clube S. V. M., Napier W. M. (1984). “The microstructure of terrestrial catastrophism”. Monthly Notices of the Royal Astronomical Society, 211, 953–968.
Drummond J. D. (1981). “A test of comet and meteor shower associations”. Icarus, 45, 545–553.
Egal A., Gural P. S., Vaubaillon J., Colas F., Thuillot W. (2017). “The challenge associated with the robust computation of meteor velocities from video and photographic records”. Icarus, 294, 43–57.
Guennoun M., Vaubaillon J., Čapek D., Koten P., Benkaldoun Z. (2019). “A robust method to identify meteor showers new parent bodies from the SonotaCo and EDMOND meteoroid orbit databases”. Astronomy & Astrophysics, 622, id. A84, 9 pp.
Hirayama K. (1918). “Groups of asteroids probably of common origin”. Astronomical Journal, 31, iss. 743, 185–188.
Jenniskens P. (2004). “2003 EH1 Is the Quadrantid Shower Parent Comet”. Astronomical Journal, 127, 3018–3022.
Jenniskens P. (2007). “The IAU Meteor Shower Nomenclature Rules”. In: Trigo-Rodríguez J.M., Rietmeijer F.J.M., Llorca J., Janches D., editors, Advances in Meteoroid and Meteor Science. Springer, New York, NY. Pages 5–6.
Jenniskens P. (2008). “The IAU Meteor Shower Nomenclature Rules”. Earth, Moon, and Planets, 102, 5–9.
Jenniskens P., Jopek T. J., Janches D., Hajduková M., Kokhirova G. I., Rudawska, R. (2020). “On removing showers from the IAU Working List of Meteor Showers”. Planetary and Space Science, 182, article id. 104821 (3 pages).
Jewitt D., Hsieh H., Agarwal J. (2015). “The Complex History of Trojan Asteroids”. In: P. Michel, F. DeMeo, W. Bottke, editors, Asteroids IV. University of Arizona Space Science Series. pages 203–220.
Jopek T. J. (1993). “Remarks on the meteor orbital similarity D-criterion”. Icarus, 106, 603–607.
Jopek T. J. and Williams I. P. (2013). “Stream and sporadic meteoroids associated with near-Earth objects”. Monthly Notices of the Royal Astronomical Society, 430, 2377–2389.
Kinoshita H., Yoshida H., Nakai H. (1991). “Symplectic integrators and their application to dynamical astronomy”. Celestial Mechanics and Dynamical Astronomy, 50, 59–71.
Micheli M., Bernardi F., Tholen D. J. (2008). “Updated analysis of the dynamical relation between asteroid 2003 EH1 and comets C/1490 Y1 and C/1385 U1”. Monthly Notices of the Royal Astronomical Society, 390, L6–L8.
Neslušan L., Svoren J., Porubčan V. (1998). “A computer program of calculation of a theoretical meteor-stream radiant”. Astronomy and Astrophysics, 331, 411–413.
Porubčan V., Williams I. P., Kornoš L. (2004). “Associations Between Asteroids and Meteoroid Streams”. Earth, Moon, and Planets, 95, 697–712.
Porubčan V., Kornoš L., Williams I. P. (2006). “The Taurid complex meteor showers and asteroids”. Contributions of the Astronomical Observatory Skalnate Pleso, 36, 103–117.
Rein H., Liu S.-F. (2012). “REBOUND: An open-source multi-purpose N-body code for collisional dynamics”. Astronomy and Astrophysics, 537, 10 pages. 10.1051/0004-6361/201118085.
Rein H., Tamayo D. (2015). “WHFAST: a fast and unbiased implementation of a symplectic Wisdom–Holman integrator for long-term gravitational simulations”. Monthly Notices of the Royal Astronomical Society, 452, 376–388.
Rudawska R., Vaubaillon J., Atreya P. (2012). “Association of individual meteors with their parent bodies”. Astronomy & Astrophysics, 541, id. A2, 5 pp.
Schiaparelli G. V. (1867a). “Sur la relation qui existe entre les Comètes et les Etoiles filantes”. Monthly Notices of the Royal Astronomical Society, 27, 246–247.
Schiaparelli G. V. (1867b). “Sur la relation qui existe entre les comètes et les étoiles filantes”. Astronomische Nachrichten, 68, 331–332.
Šegon D., Gural P., Andreić Ž., Skokić I., Korlević K., Vida D., Novoselnik F. (2014). “A parent body search across several video meteor data bases”. In: T.J. Jopek, F.J.M. Rietmeijer, J. Watanabe, I.P. Williams, editors, Meteoroids 2013, Proceedings of the Astronomical Conference held at A.M. University, Poznań, Poland, Aug. 26-30, 2013. A.M. University Press, pages 251–262.
Southworth R. R. and Hawkins G. S. (1963). “Statistics of meteor streams”. Smithson. Contrib. Astrophys., 7, 261–286.
Svoren J, Neslušan L., Porubčan V. (1993). “Applicability of meteor radiant determination methods depending on orbit type. I. High-eccentric orbits”. Contributions of the Astronomical Observatory Skalnate Pleso, 23, 23–44.
Svoren J, Neslušan L., Porubčan V. (1994). “Applicability of meteor radiant determination methods depending on orbit type. II. Low-eccentric orbits”. Contributions of the Astronomical Observatory Skalnate Pleso, 24, 5–18.
Vida D., Brown P. G., Campbell-Brown M. (2018). “Modelling the measurement accuracy of pre-atmosphere velocities of meteoroids”. Monthly Notices of the Royal Astronomical Society, 479, 4307–4319.
Vida D., Gural P. S., Brown P. G., Campbell-Brown M., Wiegert P. (2019). “Estimating trajectories of meteors: an observational Monte Carlo approach–I theory”. Monthly Notices of the Royal Astronomical Society, 491, 2688–2705.
Weiss E. (1868). “Beiträge zur Kenntniss der Sternschnuppen”. Astronomische Nachrichten, 72, 81–102.
Whipple F. L., El-Din Hamid S. (1952). “On the Origin of the Taurid Meteor Streams”. Helwan Institute of Astronomy and Geophysics Bulletins. 41. 3–30.
Whipple F. L. (1983). “1983 TB and the Geminid Meteors”. IAU Circ., No. 3881, #1 (1983). Edited by Marsden B. G.
Williams I. P., Ryabova G. O., Baturin A. P. and Chernitsov A. M. (2004). “The parent of the Quadrantid meteoroid stream and asteroid 2003 EH1”. Monthly Notices of the Royal Astronomical Society, 355, 1171–1181.
Williams I. P., Jones D. C. (2007). “How useful is the ‘mean stream’ in discussing meteoroid stream evolution?” Monthly Notices of the Royal Astronomical Society, 375, 593–603.
Yeomans D. K., Yau K. K., Weissman P. R. (1996). “The impending appearance of comet Tempel-Tuttle and the Leonid meteors”. Icarus, 124, 407–413.
Zurita M., Damiglê R., Di Pietro C., Trindade L., Silva G. G., Lima A., Mota A., Arthur R. and Betzler A. S. (2019). “A bright fireball over the coast of the state of Bahia”. Boletim da Sociedade Astronômica Brasileira, 31:1, 14–16.