Abstract: Low-light video observations show a compact but weakly active meteor shower with a radiant situated in the constellation of Corvus around February 16 in recent years. This newly identified shower has been listed in the IAU Working List of Meteor Showers as the February alpha Corvids (FAC #1101). The meteoroid stream responsible is in the orbit of an unknown long-period comet that passed close to Earth’s orbit in a prior return to the inner solar system.
1 Introduction
A weak but unusually compact meteor shower (Figure 1) was added to the IAU Working List of Meteor Showers, now called the February alpha Corvids, and given the code FAC and the number 1101 (Jenniskens, 2021). The shower is significant because it marks the orbit of an unknown long-period comet that passed close to Earth’s orbit in a prior return. If part of the nucleus survived when the stream was created, it might now be a potentially hazardous comet when it returns to the inner solar system.
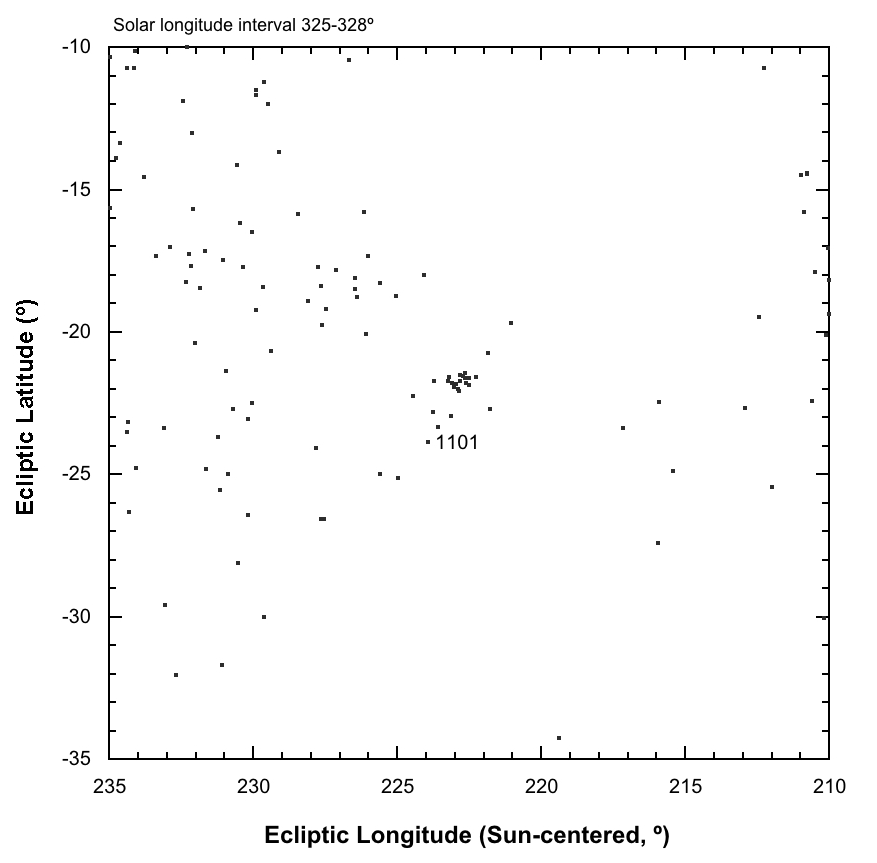
Figure 1 – The compact February alpha Corvid radiant.
2 2021 observations
On 2021 February 16, two southern-hemisphere “Cameras for Allsky Meteor Surveillance” (CAMS) networks detected and triangulated three meteors from this shower: CAMS Australia (M. Towner, Curtin University, with support of L. Toms and C. Redford) and CAMS New Zealand (J. Baggaley, University of Christchurch, with support from N. Frost, I. Crumpton, and L. and C. Duncan). The radiant was found to be located at R.A. = 180.36 ± 0.13°, Decl. = –23.81 ± 0.15° (equinox J2000.0) with geocentric velocities 54.2 ± 1.0 km/s during the solar longitude interval 327.33 – 327.53° (equinox J2000.0). Another two February alpha Corvids were detected outside this interval during February 14–19 (Jenniskens, 2021).
3 Earlier observations
This radiant was only recognized when data were compared to those from prior years. In 2020, seven meteors were triangulated by CAMS Florida (A. Howell) and CAMS California from the same radiant at R.A. = 179.72 ± 0.33°, Decl. = –23.65 ± 0.31°, with geocentric velocity 55.9 ± 1.3 km/s during the solar longitude interval 326.7–327.6° for the date of 2020 February 16. Orbital elements cover both bound and unbound orbits due to uncertainties in the measured velocities. The median orbit of all available CAMS data is slightly hyperbolic (Table 1). A geocentric velocity of vg = 54.73 ± 0.02 km/s results in a parabolic orbit with corresponding orbital elements q = 0.236 ± 0.002 AU, e = 1.0, i = 102.29 ± 0.08 deg, Peri. = 121.5 ± 0.3 deg, and Node = 146.8 ± 1.1 deg (equinox J2000.0).
4 An independent search
Because the radiant is so compact, this weak shower can be recognized also in other low-light video data back to 2011. Using the parabolic orbit above, Paul Roggemans searched in a dataset with 191,393 orbits collected by the Global Meteor Network and a dataset with 630,402 UFO-software orbits (EDMOND and SonotaCo). This search resulted in 20 similar orbits registered during the time interval λʘ [318.8°, 331.6°] or February 7–20 from a Sun-centered radiant in geocentric ecliptic coordinates at λg-λʘ [221.7°, 226.9°] and βg [–23.9°, –18.1°] within a geocentric velocity range vg = [51.6 km/s, 57.6 km/s]. Omitting 4 outliers the mean orbits for UFO software and for Global Meteor Network orbits were calculated using the method described by Jopek et al. (2006). The results are compared in Table 1 and are in good agreement with the CAMS data.
Table 1 – Median orbital elements (equinox J2000.0) and 1-sigma dispersions measured by CAMS compared with the mean values obtained from UFO software orbits and Global Meteor Network (GMN) orbits (the latter two courtesy of Paul Roggemans).
| CAMS | UFO-Orbit | GMN | |
| λʘ (°) | 326.8 | 324.4 | 324.8 |
| αg (°) | 179.8 ± 1.2 | 178.7 | 182.3 |
| δg (°) | –23.6 ± 0.5 | –23.1 | –22.0 |
| vg (km/s) | 55.0 ± 1.1 | 54.5 | 55.4 |
| λ-λʘ (°) | 222.8 ± 0.3 | 223.9 | 226.3 |
| β (°) | -21.7 ± 0.2 | –21.4 | –19.3 |
| a (AU) | -.- | 10.0 | 6.2 |
| q (AU) | 0.241 ± 0.011 | 0.229 | 0.224 |
| e | 1.009 ± 0.022 | 0.977 | 0.964 |
| ω (°) | 120.5 ± 2.6 | 123.3 | 124.8 |
| Ω (°) | 147.0 ± 1.2 | 144.1 | 146.0 |
| i (°) | 102.7 ± 1.5 | 104.7 | 111.6 |
| Π (°) | 268.1 ± 3.3 | 267.4 | 270.7 |
| Q (AU) | -.- | 19.7 | 12.2 |
| Tj | -0.34 | 0.37 | 0.62 |
| P (y) | -.- | 31.5 | 15.5 |
| N | 15 | 10 | 6 |
Acknowledgement: I thank Paul Roggemans for providing this confirmation from independent low-light video observations.
References
Jenniskens P. (2021). “February alpha Corvid meteors”. CBET 4954 edited by Daniel W. E. Green, published 2021 April 7.
Jopek T. J., Rudawska R., and Pretka-Ziomek H. (2006). “Calculation of the mean orbit of a meteoroid stream”. Monthly Notices of the Royal Astronomical Society, 371, 1367–1372.